|
Subtracting Fractions
Loading
If you’ve seen our adding fractions page, then you should know that the story about subtracting is pretty much the same. It has the same preconditions. In order to subtract any fractions their denominators must be alike. If they’re not - we have to make them alike by expanding or simplifying fractions. We do that by finding a LCD (least common denominator) for our denominators, then multiplying each to reach that LCD. Since we try to do things gradually – we’re going to start with easy examples and build our way to more complicated ones.
So we’ll cover these cases:
Subtracting Fractions
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
 |
 |
 |
||||||||||
| 2 | − | 1 | = | 2-1 | = | 1 | ||||||
| 2 | 2 | 2 | 2 | |||||||||
So, we had one whole pizza cut in two. We ate one half, and what’s left is the other half.
The red images really speak for themselves, but always combine them with numbers below. The images are just crutches, but soon you’ll be able to walk without them.
Think of a denominator being just the size, or dimension of slices. So we’re dealing with number of slices (numerators) provided we have the same size of slices (like denominators). Since this is our case now, if we had 5 parts and ate 1, obviously we end up with 4. And since 3 thirds form 1 whole pizza like a puzzle, we say that we have 1 whole pie and one third.

 |
 |

 |

 |
|||||||||||||
| 5 | − | 1 | = | 5-1 | = | 4 | = |
1 |
1 | |||||||
| 3 | 3 | 3 | 3 | 3 | ||||||||||||
Now in this example we could say that the pizza delivery guy delivered 1 whole and 3 quarters of another. So we skip his tip, kick him in the butt, and eat one quarter. ;-)

 |
 |

 |

 |
|||||||||||||||
| 7 | − | 1 | = | 7-1 | = | 6 | = | 3 | = |
1 |
1 | |||||||
| 4 | 4 | 4 | 4 | 2 | 2 | |||||||||||||
Now we have 6/4, both even numbers, which means we can simplify dividing both the numerator and denominator at least by 2. New situation is 3 halves (improper fraction form), and finally we can write it down as 1 whole and 1 half (mixed fraction).
We’re spicing up a bit by putting bigger numerators than 1, but same numerators. You’ll se that’s not a big change actually. With our like denominators, we focusing only on numerators, almost as we are not dealing with fractions at all.
Second case of
Subtracting Fractions with Like Denominators
- Numerators > 1
- Like Denominators

 |
 |
 |
||||||||
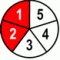
| 7 | − | 4 | = | 7-4 | = | 3 | ||||
| 5 | 5 | 5 | 5 | |||||||
See, we didn’t touch the fives. Just performed very simple subtraction to 10.
I’m sure you starting to see there is much ado about nothing in these notorious fractions. :-) Once we spotted the same denominators – we are focusing only on subtracting numerators (i.e. eating slices).
Now the numerators are a bit bigger, but no big deal. There’s little simplifying at the end.

 |

 |
 |
 |
||||||||||||
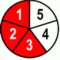
| 11 | − | 7 | = | 11-7 | = | 4 | ÷2 | = | 2 | ||||||
| 6 | 6 | 6 | 6 | ÷2 | 3 | ||||||||||
Now even more slices, but if you follow the previous examples – there’s nothing to worry about.


 |

 |

 |

 |
||||||||||||
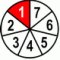
| 17 | − | 9 | = | 17-9 | = | 8 | = |
1 |
1 | ||||||
| 7 | 7 | 7 | 7 | 7 | |||||||||||
With 8/7 we crossed over a 1 whole by one seventh.
OK, enough with this easy stuff, let’s try something else.
Continue to
Subtracting fractions with unlike denominators.





