Subtracting Fractions With Unlike Denominators

Subtracting Fractions
With Unlike Denominators

Subtracting and adding of fractions with unlike denominators is all about denominators. Their stories are almost the same, only the endings differ.

You don't even look at the numerators until you make the denominators the same. We're making them the same by expanding or simplifying fractions.

In the previous lesson we covered:

Subtracting fractions with like denominators:

And now it's time to cover:

Subtracting fractions with unlike denominators:

Numerators = 1, unlike denominators
Numerators > 1, unlike denominators

Since now we’re learning subtracting fractions with unlike denominators, let’s take our numerators out of the picture for a moment and see what happens.

First case of
Subtracting Fractions with Unlike Denominators

Numerators = 1
Unlike denominators

Evidently we can’t avoid expanding and simplifying fractions whenever different denominators appear. So we have to expand our first fraction to get the same denominators.


1	×2	−	1	=	2	−	1	=	1
4	×2	−	8	=	8	−	8	=	8

Visually it’s obvious that a fourth is twice as big as eighths. But note this, I didn’t say that a 4 is bigger than an 8. Let me elaborate.

When you see an 8 and a 4 in the denominator place, you might wonder how can a bigger number be smaller than a smaller one. But that’s why this is not an 8 and a 4, but an eighth (of a whole), therefore smaller than a fourth. I hope I didn’t make things worse with this "genius" elaboration. ;-)

In this next example we expand both of our fractions to meet the necessary same denominators criteria for adding and subtracting fractions with unlike denominators.

Since 2 and 7 are prime numbers (can’t be factored by any number except for 1 and itself), finding LCD is easy – we multiply them and get 14. So we’re transforming our halves and sevenths into 14ths by multiplying both numerator and denominator with missing-to-meet-14 factor. :-)
After that, it’s easy peasy.


1	×7	−	1	×2	=	7	−	2	=	5
2	×7	−	7	×2	=	14	−	14	=	14

Here’s another idea. Subtracting can also be representing as counting down, so if we count down 2 from 7 we get 5 (although this tactic is not so good for bigger numbers).

This "equalizing" denominators is very important, so let’s play some more.

In this situation we again have two prime numbers for denominators, so automatically we know that our LCD is the product of those two. So we’re cutting our third to 5 equal parts, and our fifth to 3 equal parts – and we have our 15ths. Afterwards the same pattern – subtracting numerators.


1	×5	−	1	×3	=	5	−	3	=	2
3	×5	−	5	×3	=	15	−	15	=	15

Now we remove the "crutches". Let’s try now without images. You can imagine they’re there if you like, but don’t worry, you should do fine without them.

In the following case we have 6ths and 15ths.

1	×5	−	1	×2	=	5	−	2	=	3	÷3	=	1
6	×5	−	15	×2	=	30	−	30	=	30	÷3	=	10

At the end we did some reducing by 3.

How did we do that? How to know with what numbers should we expand our fractions? Well, since this whole story is about subtracting fractions with unlike denominators, our first step as always is finding the LCD. So let’s focus on it for a moment.

Finding an LCD
before subtracting fractions with unlike denominators

First we’re looking for as many common factors as we can for our denominators,
then we multiply those "commons" with all other "uncommon" remaining factors.

Since 6 and 15 are not prime numbers – they can be factored (i.e. represented as multiples of factors).

Here’s how:

Numbers 6 and 15 can be factored as:

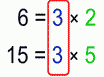
$LCD for 6 and 15$

First factor, number 3, is common both for 6 and 15 - so it’s first to go into LCD.

The next vertical pair of numbers are 2 and 5, and since those two are primes, their LCD is their product 2 × 5 = 10.

So finally, our LCD for 6 and 15 is a product of a common factor 3 and a product of different factors 2 × 5, i.e.

LCD (6,15) = 3 × 2 × 5 = 30

To "upgrade" our denominators to LCD, we multiply (expand) them by the "uncommon" factor(s) they don’t have (forget about the blue ones).

3 × 2 = 6, we haven’t used 5 – so to get to 30 we need to multiply 6 by 5

3 × 5 = 15, we haven’t used 2 – so to get to 30 we need to multiply 15 by 2

Now even more "serious" example.

1	×7	−	1	×5	=	7	−	5	=	2	÷2	=	1
30	×7	−	42	×5	=	210	−	210	=	210	÷2	=	105

The LCD in this case is 210. Simple subtraction afterwards, and again at the end, two even numbers that we can reduce (at least once) by 2.

I hear you yelling:
"Hey wise guy! You’re not going to get away with this! How the heck did you get 210 out of 30 and 42?!" :-/

Ok, ok! Hold your horses! I was going to explain the least common denominator story again. :-)

We’re looking for a (lowest) number that has all the factors of both numbers.

It’s like looking for "individual" LCDs for the factor pairs. First for common ones, then for different ones. Then we multiply them all to get our final LCD. OK? Sorry, let’s see an example. :-)

Numbers 30 and 42 can be factored as:

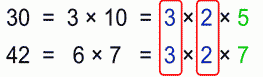
$LCD for 30 and 42$

First two factors of both numbers are 3 and 2, they are both common for 30 and 42 - so they're first to go into LCD.

The next vertical pair of numbers are 5 and 7, and since those two are primes (can't be factored), their LCD is their product 5 × 7 = 35.

So finally, our LCD for 30 and 42 is a product of common factors 3 × 2 and a product of different factors 5 × 7, i.e.

LCD (30,42) = 3 × 2 × 5 × 7 = 210

3 × 2 × 5 = 30, we haven’t used 7 – so to get to 210 we need to multiply 30 by 7

3 × 2 × 7 = 42, we haven’t used 5 – so to get to 210 we need to multiply 42 by 5

NOTE: Btw, the product of blue numbers gives us the GCF (greatest common factor) for 30 and 42.

If you need more help with LCD contact me and I’ll make a page especially for that.

Now let’s proceed to more complicated cases.

This is the real deal, but don’t worry, if you figured out the previous cases, you’ll be ok.

Second case of
Subtracting Fractions with Unlike Denominators

Numerators > 1
Unlike denominators

We apply everything we’ve learned before. We find the least common denominator, then we subtract the numerators, then at the end we reduce if necessary.

Please make sure that you pay attention on both pie images and numbers. It will deepen your understanding of subtracting fractions, as well as fractions in general.


3	×3	−	2	×2	=	9	−	8	=	1
4	×3	−	3	×2	=	12	−	12	=	12

In this second case 9 is the LCD for 9 and 3, so we’re "complicating" only the first fraction in order to get 9 by multiplying "up and down" by 3.


5	×3	−	4	=	15	−	4	=	11	=	1	2
3	×3	−	9	=	9	−	9	=	9	=	1	9

At the end we wrote it down in mixed fraction form.

The next subtracting fractions with unlike denominators case is an interesting one if you’re still struggling with what’s numerator and denominator.

Bottom line: All adding and subtracting fractions with unlike denominators "problems" start and end with finding an LCD for the denominators, so I advise you to master this skill.

In this case the (lowest) number that has 2 and 3 for factors is 6. Then what follows is easy, so I won’t waste your eye sight. :-)


3	×3	−	2	×2	=	9	−	4	=	5
2	×3	−	3	×2	=	6	−	6	=	6

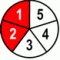
Or in this case, the lowest number that has 2 and 5 for factors is 10. So you see, this subtracting with unlike denominators business is not such a big deal. :-)

		−			=		−		=		=
5	×5	−	7	×2	=	25	−	14	=	11	=	1	1
2	×5	−	5	×2	=	10	−	10	=	10	=	1	10

Let’s kick out the help images, and try to fly solo on this subtracting fractions with unlike denominators plane.

5	×4	−	6	×3	=	20	−	18	=	2	÷2	=	1
9	×4	−	12	×3	=	36	−	36	=	36	÷2	=	18

There’s a short version of finding a LCD for 9 and 12:

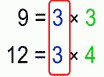
$LCD for 30 and 42$

LCD (9,12) = 3 × 3 × 4 = 36

3 × 3 = 9, we haven’t used 4 – so to get to 36 we need to multiply 9 by 4

3 × 4 = 12, we haven’t used 3 – so to get to 36 we need to multiply 12 by 3

(Again, the product of blue numbers gives us the GCF (greatest common factor) for 9 and 12.)

We could further factor number 4 to 2 × 2, but there’s no need since 3 and 4 doesn’t have any common factors.

Let’s have one more subtracting fractions with unlike denominators "pizza-less" example. :-)

14	×3	−	17	×2	=	42	−	34	=	8	÷8	=	1
24	×3	−	24	×2	=	72	−	72	=	72	÷8	=	9

This is how you get 72:

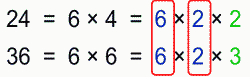
$LCD for 24 and 36$

LCD (24,36) = 6 × 2 × 2 × 3 = 72

6 × 2 × 2 = 24, we haven’t used 3 – so to get to 72 we need to multiply 24 by 3

6 × 2 × 3 = 36, we haven’t used 2 – so to get to 72 we need to multiply 36 by 2

(And again, the product of blue numbers gives us the GCF (greatest common factor) for 24 and 36.)

We could further factor number 6 to 3×2, but there’s no need since it is clearly the common factor to 24 and 36, and that’s our first goal – finding as many common factors.

So at the end of this subtracting fractions with unlike denominators page – let’s conclude and present the necessary steps to subtract fractions with different denominators.

Conclusion
for subtracting fractions with unlike denominators page: